Five-pointed star. How to draw her quickly and easily

2024 Author: Leah Sherlock | [email protected]. Last modified: 2023-12-17 05:25

The five-pointed star is the most revered symbol of all peoples of the world at all times. Her images were found at the very beginnings of civilization, when writing had not yet been invented.

The very first image of a five-pointed star found by archaeologists dates back to 3500 BC. She was depicted on a clay tablet found during excavations in the Sumerian city of Uruk.

The star symbol was popular in ancient Egypt and Babylon. He was revered by the ancient Romans and Greeks, considering the five-pointed star a symbol of the cycle in nature. The Greeks associated the five corners of the star with the five elements that our world is made of - earth, water, air, fire and ether.

The five-pointed star is an attribute of the coats of arms and flags of many modern states and is present on military insignia.

But drawing this simple figure is not as easy as it might seem at first glance.

How to draw a five-pointed star without taking your pencil off the paper

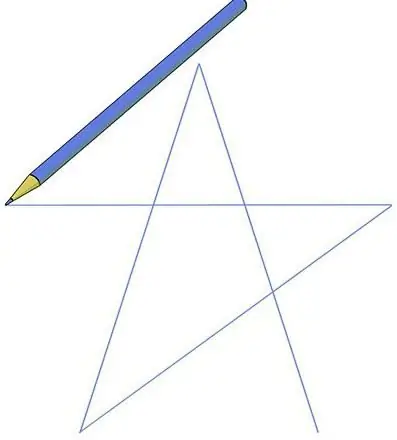
The great Greek thinker and mathematician Pythagoras called the five-pointed star mathematical perfection. Indeed, thisa complex figure can be drawn with one broken line, without lifting the pencil from the sheet of paper and returning at the end to the same starting point from which the drawing was started.

Here it is, a complex and simple figure at first glance - a five-pointed star. How to draw it with one broken line, you can see from the picture.

How to draw a star using a ruler and a protractor

Now we will learn how to draw the correct five-pointed star. From measuring instruments you will need a ruler and a protractor.

To make a star, you need to draw segments of the same length so that the internal angles between all five vertices of the figure are equal to 36°. In practice, this is done as follows - an angle of 36 ° is drawn, segments of the same length are measured from its vertex, and new straight lines are drawn from their end points at an angle of 36 °.

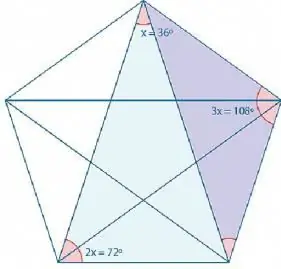
You can also approach the problem in a slightly different way by drawing an equilateral pentagon with 106° corner vertices, and then connecting its opposite corners with line segments.

If you follow all the conditions, you will end up with a beautiful five-pointed star. How to draw her in an easier way, read on.

How to draw a star with a compass and protractor

Now you need a compass and a protractor. Since there are 360 ° in a circle, then in 1/5 of its part - 72 ° (360: 5 \u003d 72). Let's start building.

Draw a circle with a compass. Mark on it the starting point - the top of the star and the middle of the circle. Take a protractor, align its center with the center of the circle, and along the entire length of the circle, mark the future points of the star's vertices with risks every 72 °.

It remains to connect them with straight lines, and you get a beautiful five-pointed star. How to draw it if there is no protractor at hand? You can do without it, the only tools you need are a ruler and a compass.

How to draw a star with a compass and ruler

How to draw a five-pointed star with a compass? Consider option 1.

Draw a circle. We measure its diameter with a ruler. We perform simple mathematical operations: we multiply the diameter of the circle by a factor of 0.58779. The result is the required length of the chord (a straight line connecting 2 points of a curved line, and in our case, a circle), with which we can divide the circle into 5 equal parts.

For example, the diameter of a circle is 7 cm. Multiply 7 x 0.58779=4.11453, round to tenths (since it is not possible to draw a segment of a more accurate length on paper), we get 4.1 cm. This and will be the desired chord length.

It remains to move apart and fix the legs of the compass by this amount, and you can make notches on the circle. When you connect them, you will get a five-pointed star.

How to draw a figure in a different way? Consider option 2.

First, let's draw a regular pentagon inscribed in a circle. How to draw correcta five-pointed star based on it, shown in Figure 2.

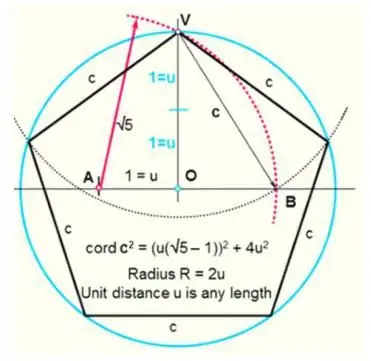
Draw a circle with a compass. Let's conditionally designate its center as O. Draw a straight line through the point O - the diameter of our circle. Draw the radius of this circle so that it is perpendicular to the diameter. Let's denote the intersection point of the radius with the circle as V. To the left of point O, set aside a distance equal to half the length of the radius of this circle, mark it as point A. From point A through point V, draw a semicircle until it intersects with the diameter line (in the figure it is highlighted in red) and mark it with point B. The length of the segment VB will be the length of the chord, with the help of which the circle is divided into 5 equal parts. It remains only to connect the obtained points in the form of a star.